You will likely be familiar with constrained wave from wind turbine standards such as IEC61400-3-1 or DNV-ST-0437.

As in DNV-ST-0437

‘Simulations shorter than 1 hour may be applied for the estimation of extreme events if this does not compromise extreme load statistics, e.g., six 10-min simulations. Constrained wave methods may be used in this case.’

In brief, constrained wave is a made-up wave train that embeds a nonlinear regular wave to a linear irregular wave train. It is used in load simulations of offshore wind turbines, to provide more realistic waves yet allow reduced simulation time. As an illustration, the image (a) below shows an example constrained wave, where the nonlinear regular wave (orange line) is inserted in the linear irregular wave (blue line) in the image (c) below.

Why we need constrained waves

Wind turbine design relies on the results of load simulations. As offshore wind turbines are highly dynamic, its load simulations need to consider both the stochastic nature and the nonlinear nature of the waves. See IEC for more information on this. However, this is not satisfied by common wave theories. For example, regular waves can be nonlinear but they are deterministic (non-stochastic), while irregular waves are stochastic and linear. Therefore, it is of great interest to have a wave train that is both nonlinear and stochastic. To this end, constrained waves are developed. It is a wave train that embeds one nonlinear regular wave into a series of irregular linear waves, hence accounts for both the stochasticity and the nonlinearity.

How to generate constrained waves

As regular waves can be be nonlinear, and irregular waves are stochastic, one intuitive idea is to blend the two wave types, generating a wave that is both nonlinear and stochastic. Following this line, a straightforward methodology is to ‘cut and paste’; we can cut the regular wave and paste it to suitable positions in the irregular waves. A suitable position is where the troughs of the irregular waves are equal or close to that of the regular wave, so that they can be blended smoothly. There are two approaches to achieve that.

Approach 1: Searching

This method uses the following steps:

1. A nonlinear regular wave is generated first to ascertain the elevation of the troughs, as shown in image a.

2. Then a time history of the irregular wave is searched for a trough elevation that was close to the obtained trough elevation of the nonlinear wave. A suitable position is marked by a red cross in image b. An error tolerance of the trough can be applied during the searching and 1% is found to be a good trade-off between simulation time and accuracy.

3. The nonlinear regular wave is inserted in the irregular wave, as shown in image (c). The length of the generated wave train is the sum of the two wave trains.

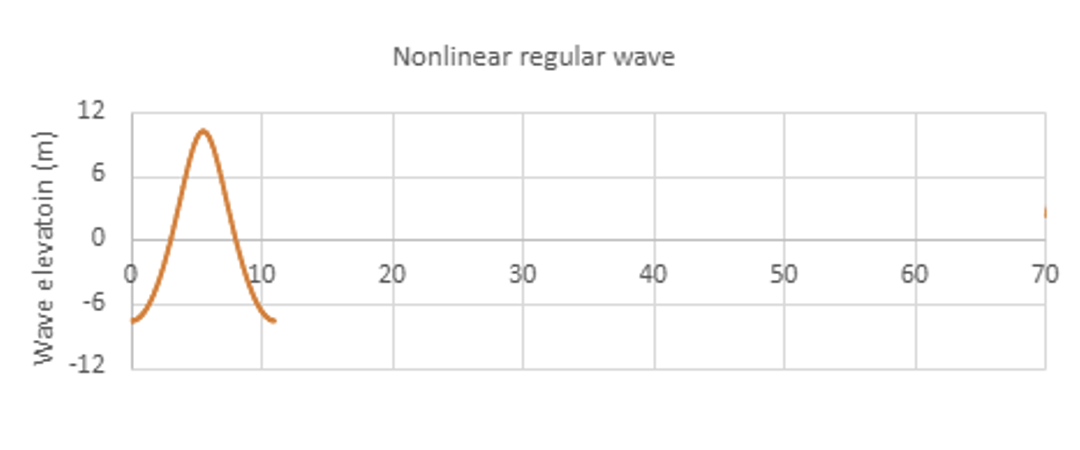
Image a.

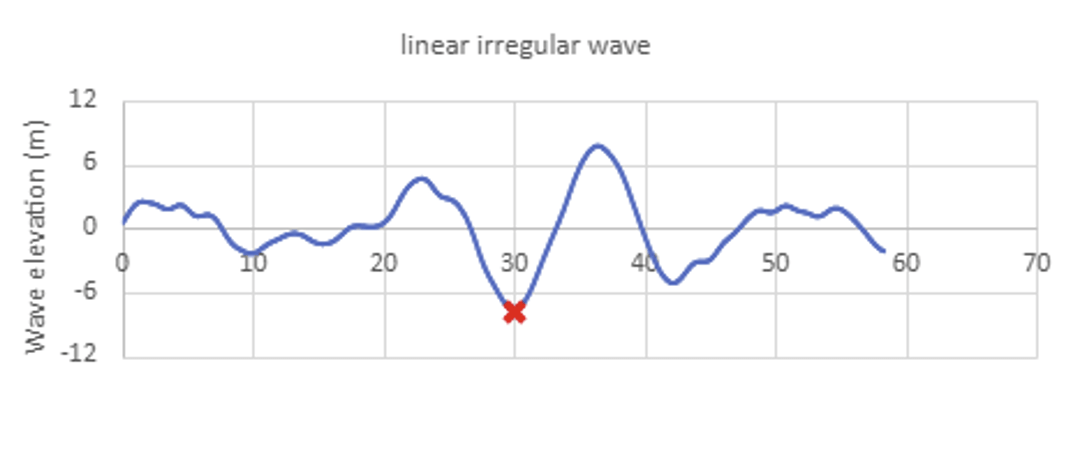
Image b.

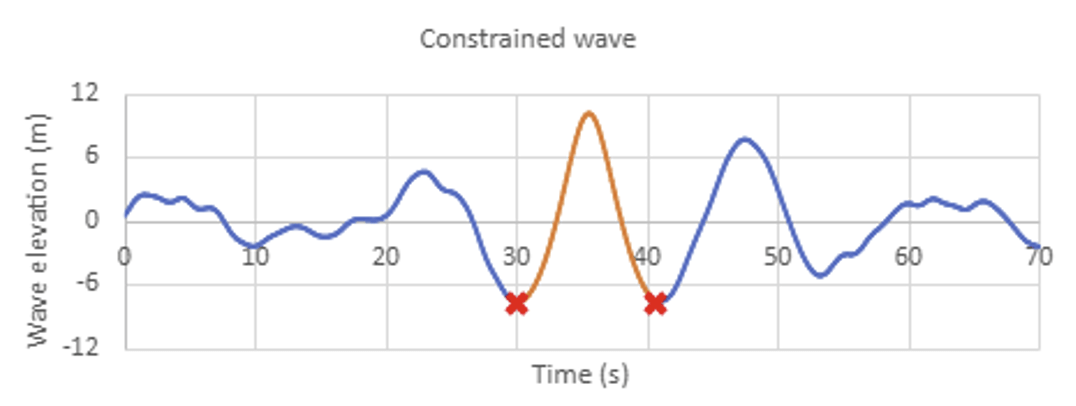
Image c.

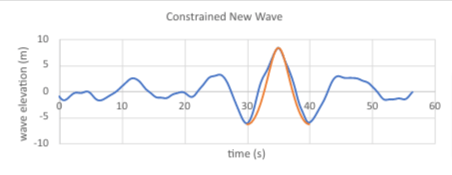
Approach 2: Constrained New Wave

This method has the following steps with a significant difference in step 2.

1. A nonlinear regular wave is generated first to ascertain the elevation of the troughs, as shown in image (d).

2. ‘Constraining’ a linear wave train so that a peak and its neighbouring troughs appear in suitable positions, as indicated by the black bars in image (e). This is achieved by seeing the irregular waves as a Gaussian process. More details are available from Rainey and Camp.

3. At the two points marked by the red crosses in image (f), the irregular wave segment is replaced with the nonlinear regular wave. Image (f) shows the generated constrained wave.

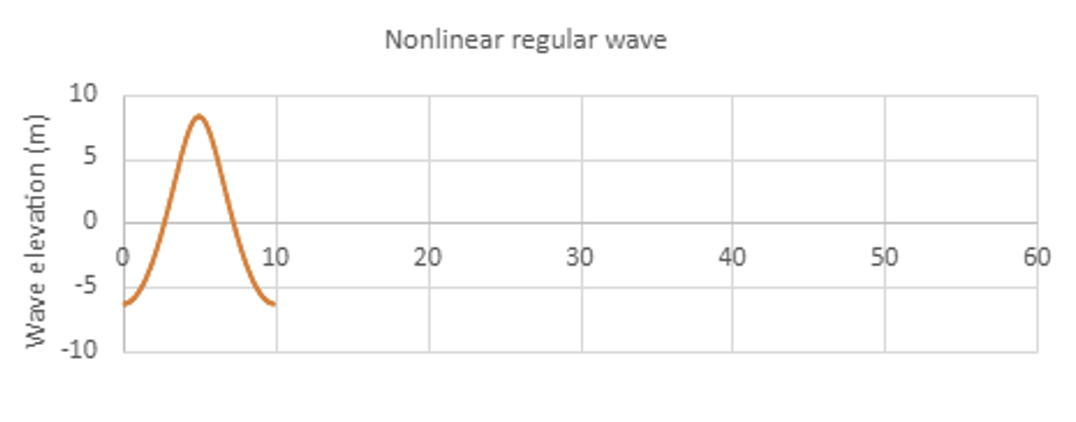
Image d.

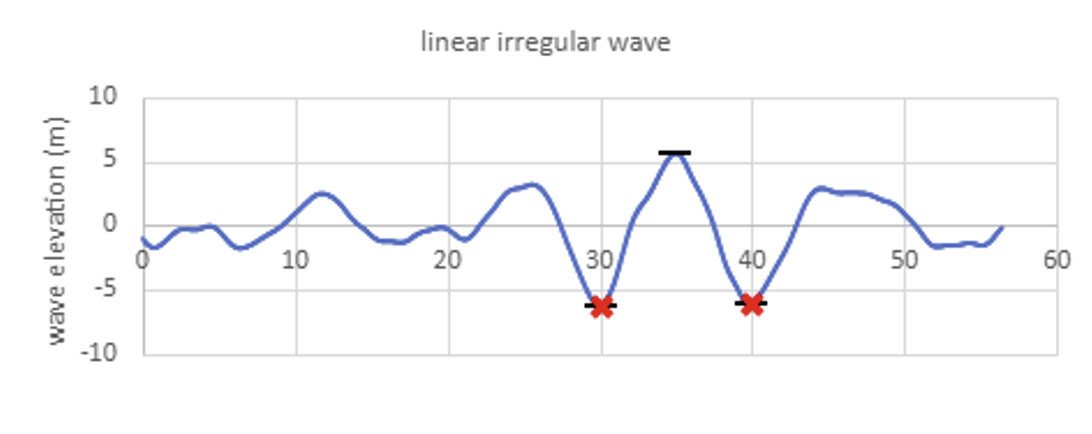
Image e.

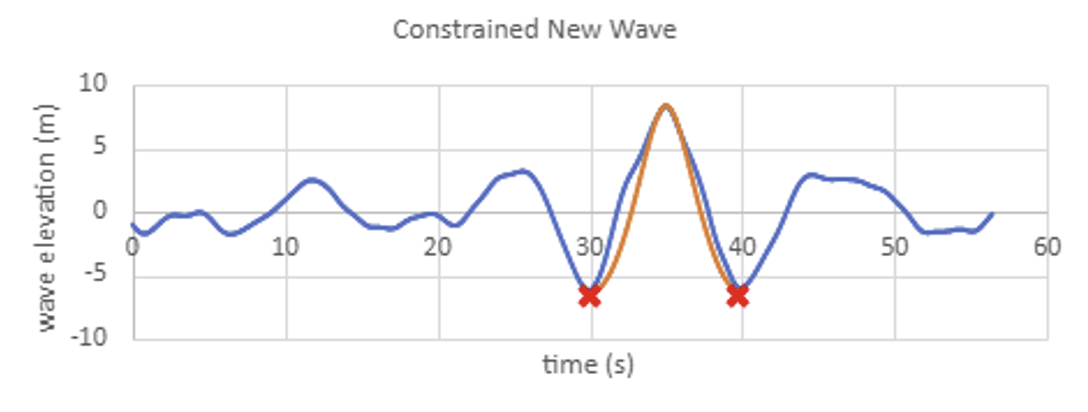
Image f.

Discussion points

Reduced simulation time. As offshore wind turbines are highly dynamic, there are hundreds of design load cases for ultimate limit state to consider. Each design load case requires a simulation time of at least one hour so that it can fully represent the possible sea state. Such a simulation task requires huge computational resources and slows down the design iteration. This can be mitigated by applying constrained waves, which allow reduced simulation time. This is because the constrained wave ensures the occurrence of an extreme wave in a small and fixed period of wave simulation. The simulation time is allowed to be reduced to 10 minutes, as stated in the quote at the beginning of this article.

Weighting function. In both approaches, the nonlinear regular waves are ‘hard’ connected to the irregular wave. That means there are small discontinuities at the points where regular wave and irregular waves are connected. The discontinuity can be eliminated by introducing a weighting function to the blending region so that the wave changes smoothly across the two wave types. Further information on weighting functions can be found in Rainey and Camp.

The pros and cons of the two methods

The searching method is straightforward to understand and implement. But it is computationally inefficient to find the suitable position because long simulations are typically required before a suitably large wave appears ‘by chance’.

This is why the constrained New Wave method was proposed. Constrained New Wave is able to find the suitable positions in a limited and constant time. However, it takes time to understand the theoretical part, especially without background knowledge on stochastic process.

Empire specialists can effectively and efficiently assist with your offshore wind project. To find out more, please get in touch with the team at Empire Engineering.